This comprehensive guide will equip you with all the essential knowledge and strategies to excel in one of the most challenging subjects in the Western Australian Certificate of Education (WACE) curriculum.

Mathematics Methods is a crucial subject for many university pathways, and performing well can significantly boost your ATAR. Whether you're aiming for a top score or just want to improve your understanding, this article will cover everything from exam format and revision techniques to common pitfalls and last-minute tips.

Summary of Units

Below we will cover Units 3 and 4 in  Year 12 - Mathematics Methods and all the sub-topics you will need to understand to do well on your Mathematics Methods exam:

Unit 3

The study of calculus covers derivatives of exponential and trigonometric functions, differentiation techniques, and their applications, including optimisation and graph sketching. It also includes integration, focusing on its role as the reverse of differentiation and in calculating areas, with emphasis on the fundamental theorem of calculus. In statistics, the introduction of discrete random variables and their use in modelling random processes leads to the development of a framework for statistical inference. Technology is assumed to be available for computational support.

Topic 3.1: Further differentiation and applications (20 hours)

Exponential functions

Trigonometric functions

Differentiation rules

The second derivative and applications of differentiation

Topic 3.2: Integrals (20 hours)

Anti-differentiation

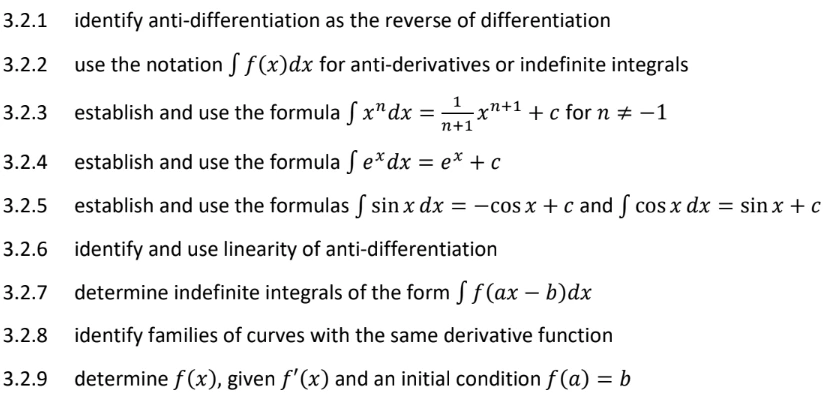
Definite integrals

Fundamental theorem

Applications of integration

Topic 3.3: Discrete random variables (15 hours)

General discrete random variables

3.3.1 develop the concepts of a discrete random variable and its associated probability function, and their use in modelling data
‍3.3.2 use relative frequencies obtained from data to obtain point estimates of probabilities associated with a discrete random variable
‍3.3.3 identify uniform discrete random variables and use them to model random phenomena with equally likely outcomes
‍3.3.4 examine simple examples of non-uniform discrete random variables
‍3.3.5 identify the mean or expected value of a discrete random variable as a measurement of centre, and evaluate it in simple cases
‍3.3.6 identify the variance and standard deviation of a discrete random variable as measures of spread, and evaluate them using technology
‍3.3.7 examine the effects of linear changes of scale and origin on the mean and the standard deviation
‍3.3.8 use discrete random variables and associated probabilities to solve practical problems

Bernoulli distributions

3.3.9 use a Bernoulli random variable as a model for two-outcome situations
‍3.3.10 identify contexts suitable for modelling by Bernoulli random variables
3.3.11 determine the mean p and variance p(1 − p)of the Bernoulli distribution with parameter p
‍3.3.12 use Bernoulli random variables and associated probabilities to model data and solve practical problems

Binomial distributions

Unit 4

This unit focuses on advanced mathematical concepts across calculus and statistics. It begins with the study of logarithmic function derivatives. The course then transitions to probability theory, introducing continuous random variables and their practical applications. Special attention is given to the normal distribution and its diverse uses.

The unit culminates in an exploration of statistical inference, a crucial aspect of statistics that aims to estimate population parameters using sample data. In this course, the scope of statistical inference is limited to estimating proportions in populations with two possible outcomes.

Throughout the unit, students are expected to have access to and utilise appropriate technology to support their computational work in these areas.

Topic 4.1: The logarithmic function (18 hours)

Logarithmic functions

Calculus of the natural logarithmic function

Topic 4.2: Continuous random variables and the normal distribution (15 hours)

General continuous random variables

4.2.1 use relative frequencies and histograms obtained from data to estimate probabilities associated with a continuous random variable
4.2.2 examine the concepts of a probability density function, cumulative distribution function, and probabilities associated with a continuous random variable given by integrals; examine simple types of continuous random variables and use them in appropriate contexts
‍4.2.3 identify the expected value, variance and standard deviation of a continuous random variable and evaluate them using technology
‍4.2.4 examine the effects of linear changes of scale and origin on the mean and the standard deviation

Normal distributions

4.2.5 identify contexts, such as naturally occurring variation, that are suitable for modelling by normal random variables
‍4.2.6 identify features of the graph of the probability density function of the normal distribution with mean μ and standard deviation σ and the use of the standard normal distribution
‍4.2.7 calculate probabilities and quantiles associated with a given normal distribution using technology, and use these to solve practical problems

Topic 4.3: Interval estimates for proportions (22 hours)

Random sampling

4.3.1 examine the concept of a random sample
‍4.3.2 discuss sources of bias in samples, and procedures to ensure randomness
‍4.3.3 use graphical displays of simulated data to investigate the variability of random samples from various types of distributions, including uniform, normal and Bernoulli

Sample proportions

Confidence intervals for proportions

💡Study tip! Organise your notes by the headers and sub-headers in the syllabus. This ensures you cover everything that could be on the exam and keeps your notes super organised.

Format of the Assessments

Additional Assessment Information:

• Teachers must develop an assessment outline for the pair of units, including all three assessment types.
• The outline must describe each task, indicate content to be assessed, and specify timing and weighting.
• Tasks should provide a representative sampling of Unit 3 and Unit 4 content.
• Appropriate validation processes are required for tasks not administered under controlled conditions.

Grading:

• Students are graded A to E, representing achievement levels from Excellent to Very low.
• Teachers prepare a ranked list and assign grades based on pre-determined standards.
• Grade descriptions and annotated work samples define these standards.
  ‍

💡Take notes efficiently and effectively using these tips!

What Does an A Look Like?

Are you curious as to what it takes to get an A? In the syllabus, the Department of Education in Western Australia outlines exactly what is required for a student to get an A in Mathematics Methods.
‍

‍

How to Do Well in Mathematics Methods

1. Understand the Syllabus

• Thoroughly review the syllabus content for Unit 3 and Unit 4
• Identify key topics and concepts you need to master

2. Organise Your Study Materials

• Collect all your notes, textbooks, and past assignments
• Create a structured study plan, allocating time for each topic

3. Practise Regularly

• Solve a variety of problems daily
• Focus on both routine and non-routine questions
• Use past WACE exam papers for practice

4. Master Use of Technology

• Practise with your CAS calculator
• Ensure you can use it efficiently for complex calculations

5. Focus on Understanding, Not Just Memorisation

• Understand the underlying concepts and principles
• Practice explaining mathematical ideas in your own words

💡 Check out these scientifically proven strategies to improve how you study!

6. Utilise Multiple Resources

• Use textbooks, online resources, and study guides
• Consider forming study groups with classmates

7. Tackle Your Weak Areas

• Identify topics you struggle with
• Allocate extra time to improve in these areas

8. Practice Time Management

• Complete timed practice exams
• Learn to allocate your time effectively in each exam section

9. Review and Reflect

• After each practice session, review your work
• Understand your mistakes and learn from them

10. Stay Healthy

• Maintain a balanced diet and regular sleep schedule
• Take breaks and exercise to keep your mind fresh

11. Seek Help When Needed

• Don't hesitate to ask your teacher for clarification
• Consider tutoring if you need extra support

Mistakes to Avoid

1. Misreading the Question
   • Always read each question carefully
   • Highlight key words and information
   • Ensure you understand what's being asked before starting your solution
2. Skipping Steps in Calculations
   • Show all your working, even for seemingly simple calculations
   • Remember that marks are often awarded for process, not just the final answer
3. Forgetting Units
   • Always include appropriate units in your final answer
   • Check that units are consistent throughout your calculations
4. Rounding Errors
   • Be mindful of rounding instructions in each question
   • Avoid premature rounding in multi-step problems
   • If no specific instruction is given, maintain an appropriate level of accuracy
5. Calculator Errors
   • Double-check your calculator inputs, especially for complex functions
   • Ensure your calculator is in the correct mode (e.g., radians vs degrees)
   • Practice using your calculator efficiently before the exam
6. Not Checking Answers
   • If time allows, review your answers
   • Check if your solution makes sense in the context of the question
7. Poor Time Management
   • Don't spend too much time on any single question
   • Ensure you attempt all questions, even if some are only partially completed
8. Misusing Formulas
   • Memorise key formulas correctly
   • Understand when and how to apply each formula
9. Overlooking Domain and Range
   • Consider the domain and range of functions, especially in calculus questions
   • Be aware of restrictions on variables (e.g., 𝑥 > 0 for logarithmic functions)
10. Neglecting to Justify Answers
    • Provide clear reasoning for your solutions, especially in higher-mark questions
    • Use appropriate mathematical language and notation in your explanations
11. Misinterpreting Graphs
    • Pay close attention to scales and labels on axes
    • Don't confuse different types of graphs (e.g., a function and its derivative)
12. Panicking Under Pressure
    • If you're stuck, move on and return to the question later if time permits
    • Remember your preparation and stay calm
13. Ignoring the Context
    • In applied problems, consider whether your answer makes sense in the real-world context
    • Be prepared to interpret mathematical results in practical terms
14. Forgetting to Use Given Information
    • Utilise all information provided in the question
    • Don't overlook important details that could simplify your solution
15. Algebraic Mistakes
    • Be careful with signs when manipulating equations
    • Double-check your algebraic simplifications

Link to Past Papers

Why Past Papers are the Best Way to Revise for WACE Mathematics Methods Exam

Past papers are an invaluable resource for students preparing for the WACE Mathematics Methods Exam. Here's why they are considered the best revision tool:

1. Familiarisation with Question Structure
   • WACE tends to use consistent question structures, which may differ from those in textbooks or other resources.
   • Regular practice with past papers helps you become comfortable with these specific structures, giving you a significant advantage during the actual exam.
2. Quick Identification of Challenging Areas
   • By working through past papers, you can quickly identify the types and content of questions you find most difficult.
   • This allows you to focus your revision efforts on these challenging areas, maximising the efficiency of your study time.
3. Time Management Practice
   • Past papers help you identify parts of the exam where you need to allocate more time.
   • Regular practice with timed conditions can improve your pacing and ensure you complete all sections of the exam.
4. Exposure to Real Exam Conditions
   • Past papers provide an authentic exam experience, helping you get accustomed to the pressure and format of the actual test.
5. Understanding Mark Allocation
   • Past papers, along with their marking schemes, show you how marks are typically allocated for different types of questions.
   • This insight can help you understand where to focus your efforts for maximum marks.
6. Tracking Progress
   • By attempting past papers over time, you can track your progress and see improvements in your speed, accuracy, and overall performance.
7. Identifying Common Themes
   • Over several years, certain themes or question styles may recur. Recognising these patterns can help you prepare more effectively.
8. Practising Application of Knowledge
   • Past papers often require you to apply your knowledge in ways that differ from standard textbook questions, enhancing your problem-solving skills.
9. Familiarity with Calculator Use
   • WACE Mathematics Methods Exam has both calculator-free and calculator-assumed sections. Past papers help you practice when and how to use your calculator effectively.
10. Understanding the Syllabus in Context
    • Past papers show how theoretical concepts from the syllabus are applied in practical exam questions.

Caution Note: When reviewing exams from several years ago, be aware that some topics may no longer be part of the current syllabus or may be asked about differently. Always cross-reference with the most recent syllabus and exam structure information provided by SCSA (School Curriculum and Standards Authority). It's advisable to focus more on recent past papers (last 3-5 years) for the most relevant practice.

Remember, while past papers are an excellent revision tool, they should be used in conjunction with other study methods for comprehensive exam preparation.

‍

WACE Mathematics Methods Exam: Preparation Tips

General Preparation Tips

1. Master Your CAS Calculator
   • Ensure you're proficient with your CAS calculator for the calculator-assumed section.
   • Practise complex calculations and graphing functions.
2. Focus on Key Areas
   • Pay special attention to calculus and statistics, as these are major themes in Mathematics Methods.
   • Review exponential, logarithmic, and trigonometric functions thoroughly.
3. Practise Both Sections
   • Allocate practice time for both calculator-free and calculator-assumed sections.
   • Develop strategies for tackling questions without a calculator.
4. Understand Mark Allocation
   • Review past papers to understand how marks are typically allocated.
   • Practice providing full working out for higher-mark questions.
5. Revise Formulae
   • While a formula sheet is provided, familiarise yourself with key formulae to save time during the exam.
6. Work on Time Management
   • Practise completing questions within time constraints.
   • Learn to allocate time based on the marks for each question.

The Night Before the Exam

1. Light Review
   • Do a light review of key concepts and formulae.
   • Avoid trying to learn new material at this stage.
2. Prepare Your Materials
   • Ensure your calculator is working and fully charged.
   • Pack extra batteries just in case.
   • Organise your pens, pencils, and other allowed materials.
3. Review Exam Structure
   • Remind yourself of the exam format, including time allocations for each section.
4. Relax and Rest
   • Engage in light, relaxing activities to calm your mind.
   • Aim for a good night's sleep (at least 8 hours if possible).

The Day of the Exam

1. Healthy Breakfast
   • Eat a nutritious breakfast to fuel your brain.
   • Stay hydrated but don't overdo it.
2. Arrive Early
   • Give yourself plenty of time to get to the exam venue.
   • Use any waiting time for light revision or relaxation techniques.
3. Warm-Up
   • Do a few simple calculations to warm up your mathematical thinking.
   • This could include basic differentiation or integration problems.
4. Read Instructions Carefully
   • Take time to read all instructions at the start of the exam.
   • Note any choice of questions or specific requirements.
5. Strategic Approach
   • Start with questions you're confident about to build momentum.
   • In the calculator-assumed section, use your CAS calculator efficiently.
6. Time Management
   • Keep an eye on the time, especially when transitioning between sections.
   • If stuck on a question, move on and return to it if time allows.
7. Show Your Working
   • Remember to show all your working, especially for high-mark questions.
   • This can help you earn partial marks even if the final answer is incorrect.
8. Stay Calm
   • If you encounter a difficult question, take a deep breath and approach it step-by-step.
   • Remember your preparation and trust in your abilities.

Remember, the WACE Mathematics Methods exam tests not just your knowledge, but also your ability to apply mathematical concepts to solve problems. Stay confident, manage your time wisely, and demonstrate your understanding through clear, logical working out.

‍

Conclusion

Remember, success comes from consistent preparation, strategic thinking, and confidence in your abilities. Start early, practice regularly, and seek help when needed. The skills you're developing will serve you well beyond this exam.

Stay focused, take care of yourself, and approach the exam with determination. You've got this!